Pink Tower of Jewels
October 9th, 2024
This post provides a glimpse into how incredibly connected, and multifaceted the Montessori math materials are, and might be a preview of what will be demonstrated during the upcoming Voyage of Discovery - Mathematics on Saturday, October 26, 2024.

Behold the iconic pink tower, a sensorial work that assists the child in developing fine and gross motor control, gradation skills, ordering skills, and so much more. Somewhere else in our well prepared primary classroom stands the elegant bead cabinet, located near other math materials. Intuitively, for the child, they know that everything is connected, even these materials, but it isn’t until Upper Elementary that they begin to understand and can calculate how related these materials are.
The bead cabinet holds space in each classroom from Primary through Upper Elementary. The cabinet contains several elements: two sets of bead chains; one set of short chains which is a series of color coded bead bars chained or linked together enough to make a square (five bead bars of five beads or (5 x 5) or 5²); one long bead chain with enough bead bars to make a cube (for example, 25 bead bars of five beads, or (5² x 5) or 5³; bead squares to compliment the short bead chains; bead cubes to compliment the long bead chains; and number tags to numerate each bead bar in the respective chains.
When the bead cabinet is introduced in primary classrooms it may only have the short chains and squares available for use. The use is for numeration, counting forward from a value (instead of always starting at one), and ultimately skip counting. As the year progresses long chains may be added to the cabinet as the children’s desire and ability to count and skip count higher numbers increases. The physical space that the long chains occupy is impressive as well, and provides the children who complete the long chains with a deep sense of accomplishment -as well as a concrete sense of what 9³ or 10³ represents in terms of a concrete quantity.
As children transition into Lower Elementary classrooms, they leave behind many of the practical life and sensorial works (including the pink tower) that filled their work cycles. But, many are often relieved to find their good friend, the bead cabinet, holding a place of prominence in the classroom. On the first day of Lower Elementary, the bead cabinet is available for use, complete with short chains, long chains, squares, cubes, and numerical tags. Practice with the materials continues in the same manner as Primary, with an emphasis on skip counting, doing mental math to add to previous sums, and beginning to memorize and internalize multiplication facts. Near the end of Lower Elementary, a complimentary material to the bead cabinet is presented; the decanomial bead box. This material is basically the bead bars of the bead cabinet unchained. The decanomial bead box contains 55 of each numeration (color) of bead bar. The direct aim of the material is to build a Pythagorean Square in several configurations.

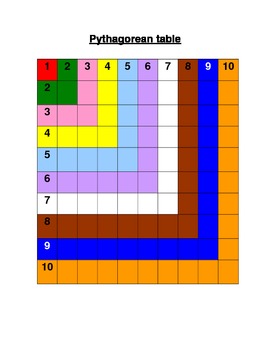
This gives the children a geometric sense of the multiplication table (here 4 x 5 has a shape as well as product) and continues (along with other materials) to solidify their math facts.
The first two passages of the decanomial are a horizontal presentation where the beads are laid out in rows (one taken one time, one taken two times, etc..) and a vertical passage where the beads are configured in columns. (Figure 1) In some classrooms, intrepid math minded children make their way through these presentations and a third presentation that is represented in Upper Elementary.
When children transition to Upper Elementary, they find many of the same math materials from their LE classroom still on the shelf next to undiscovered materials (and forgotten favorites). The bead cabinet and decanomial bead box are there to greet them. In Upper Elementary, the decanomial bead box is used as review for math facts, but also to make a connection between materials that they’ve been working with for half their lives. The final passage of the decanomial is referred to as the angular layout. (Figure 2) Here the square of a factor is placed first -diagonally from the previous square, and then the other bead bars are placed horizontally and vertically to the left and above the square respectively (2² is placed first the (2 x 1) is placed to the left of the square and (1 x 2) is place above the square).

Figure 1

Figure 2
This configuration requires borrowing higher value bead bars from a second decanomial box as the children start to discover that 64 eight (brown) bead bars are required to complete the row and column, 81 nine (blue) bead bars are needed to complete the row and column, and 100 ten (gold) bead bars to complete the final row and column. These are precisely the number of bead bars in the respective colored long bead chains of the bead cabinet. Once the angular layout of the decanomial is complete, a guide demonstrates how alternate groupings of the rows and columns of similarly colored bead bars can be combined to make squares. Surprisingly, there are 10 ten (gold) squares that can be stacked to create a 1000 cube (10³), 9 nine (blue) squares that can be stacked to make a 729 cube (9³), and so on until we’ve replaced each stack of squares with its corresponding cube from the bead cabinet. For the grand finale, each successively smaller cube is stacked on top of the previous cube to create what is known as the “tower of jewels” which is the same proportionally to our pink tower of years gone by.
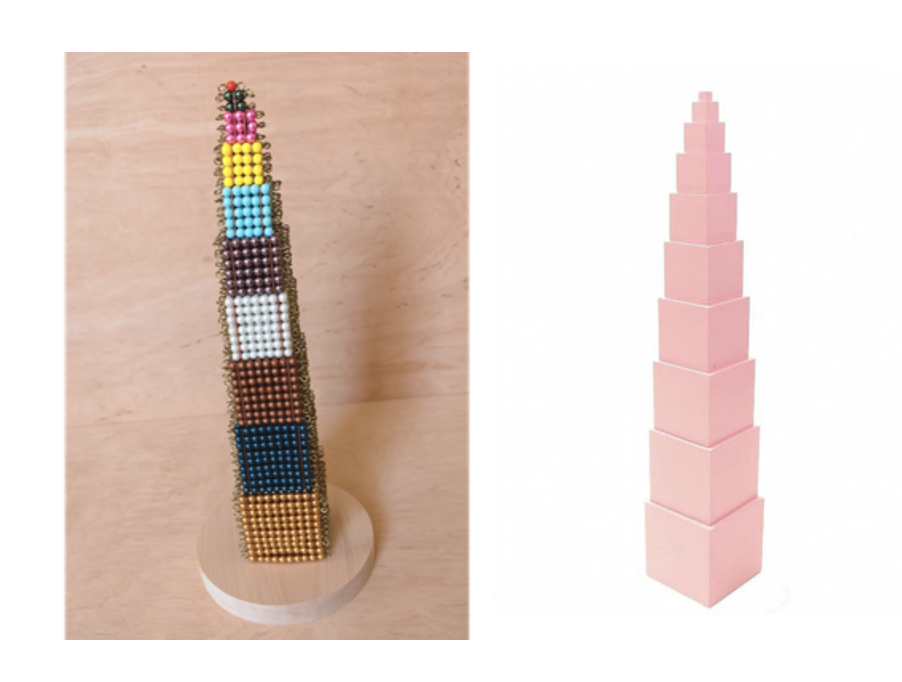
Whether this is revealed to the child by a guide or they intuitively reason a connection what is astonishing is this realization that our old friend, the bead cabinet (with us from the beginning) is a mathematical and geometric expression of the pink tower and children now have several methods for calculating the volume of the pink tower.
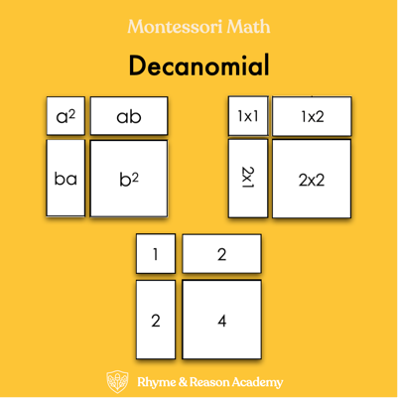
The after-bonanza of the big reveal of the tower of jewels is that there is one final articulation of the decanomial, the algebraic decanomial. Here numbers are traded for letters (variables) where a = 1, b = 2, c = 3, to j = 10. Then the children solve
(a + b + c + d + e + f + g + h + i + j)2 using the distributive property of multiplication and the angular decanomial layout as a guide.
Children leave most of these brilliant interconnected Montessori math materials behind when they move up to Middle School -because they have moved to abstraction with the aid of materials. The Middle School teachers here at WHMS have found ways to incorporate aspects of the materials (color coding) and material layout to aid children with all manner of complex calculations, for example, combing like terms in linear algebra equations. Other connections and materials are sure to be revealed at the upcoming Voyage of Discovery.

J.T. Rinker, Ph.D.
| Lead Upper Elementary Teacher |
J.T. holds a doctorate in music composition from the University of Buffalo and has worked as an adjunct professor at RPI and The College of Saint Rose and as a teacher at Morning Star Montessori School in Troy. He is credentialed through the American Montessori Society in both Lower and Upper Elementary. He first joined the Woodland Hill faculty as an intern in the Lower Elementary in 2022, and then served as an Associate Teacher in Upper Elementary. |




